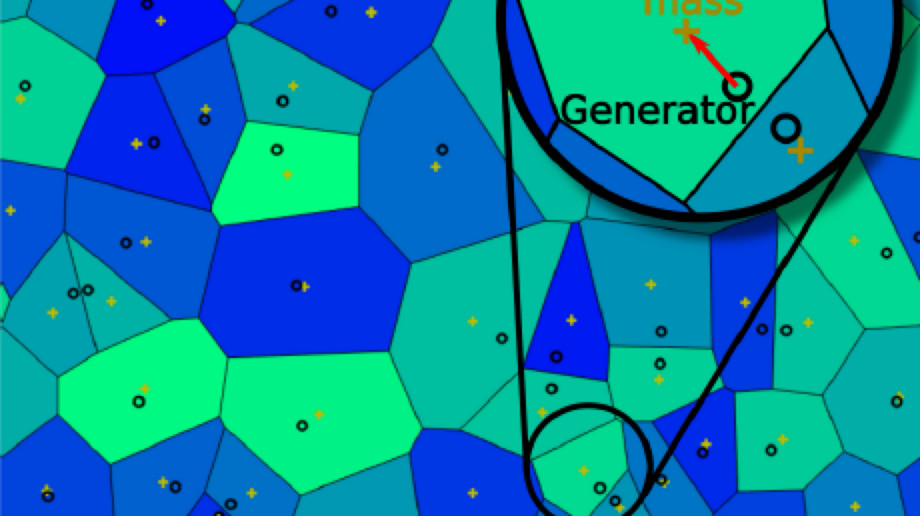
Partitions of space into cells, both ordered and disordered, play a central role in many fields of science. Two distinct principles govern their formation: The first, underlying the famous Kelvin problem, the Kepler problem and the Quantizer problem, is the question of the optimality with respect to properties such as interface area, packing density, or cell centrality. For all known such problems, the optimal solutions are crystalline, ordered structures. The second principle, which leads to disordered structures and which is far less understood, is the question whether or not dominant amorphous states exist which prevent the system from attaining the optimal solutions. A resolution of this latter question hinges on the recognition that amorphous geometries are not completely structureless. The challenge is the identification of a suitable quantitative characteristic for the degree of structural organisation. Here we consider a measure for the anomalous suppression of long-wavelength density fluctuations, known as hyperuniformity3, and show that the occurrence of disordered hyperuniform structures and the Quantizer problem are closely related. We use Lloyd’s algorithm, an algorithm for centroidal Voronoi diagrams which mimics the dynamics of soft deformable growing cell tissues. For disordered initial states, this algorithm converges to the same universal hyperuniform amorphous state, regardless of the stochastic properties of the initial state. The final universal state reveals a clear signature of the dimension of the ambient space: In 3D, the universal hyperuniform state is fully disordered without any trace of local crystalline structure. By contrast, the same procedure in 2D converges to a universal configuration that, while globally amorphous, exhibits local crystallites with a hexagonal-lattice structure. For 3D systems with energy functionals related to the Quantizer problem, such as copolymeric Frank-Kasper phases4, our results imply the likelihood of novel disordered hyperuniform phases. Due to their universality, these hyperuniform states represent a generic phase of matter in the strict sense of thermodynamics, intermediate to crystals and liquids